Converting one unit to another is something we do all the time. For example we convert quarters to dollars, cups to liters or days to weeks etc. . Many of these conversion we can do in our heads but sometimes the problems are little more difficult - a technique becomes necessary. This technique of converting from one unit to another is often called diminesional alysis. In dimensional analysis we use conversion factors to successively convert from the initial unit to the desired unit. An example of a conversion factor is 4 quaters = 1 dollar. Equivlently we can write the unit ratios (4 quater/1 dollar or 1 dollar/4 quarter). If we want to convert $120 to quarters we proceed as follows: 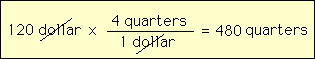 Note how the dollar is placed in the denominator to cancel the dollar in the numerator. The unit "quarters", which is what we want, is all that is left. A more difficult example might be the following: How many meters are there in a a 100 yard football field? The plan of attack would be yards -> feet -> inches -> cm -> m: 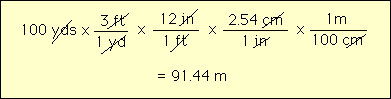 Derived units are converted in a simplar way: Suppose we want to convert 60 mph to meters/second? The plan might be miles -> feet -> inches -> cm -> m and hour -> minutes -> seconds: 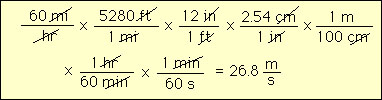 Interstingly, this result states that a car traveling at 60 mph will travel almost 27 meters every second! If we want to convert areas or volumes we must be careful keeping proper track of the squares and cubes. Suppose we want to convert a 10 cm x 12 cm x 15 cm rectangular box to cubic inches. We need to use the conversion factor 2.54 cm = 1 in in the following way: 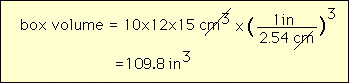 Note that the cubic centimeters cancel only because the conversion factor is cubed. Sometimes it is possible to convert between different kinds of units, e.g., mass to volume. this kind of conversion will be discussed later. |